Maths Index

Properties of Solids Shapes

C_x, C_y, C_z ...Centroid /Centre of Gravity

Point at which the entire weight of a body may be considered as concentrated so that if supported at this point the body would remain in equilibrium in any position.  If body is considered to be a particle then all of the mass or area is assumed to act at this point.

I... Mass Moment of Inertia.

The Moment of Inertia quantifies the resistance of a physical object to angular acceleration.   Moment of inertia is to rotational motion as mass is to linear motion.

In general, an object's moment of inertia depends on its shape and the distribution of mass within that shape: the greater the concentration of material away from the object's centroid, the larger the moment of inertia. The principal moments of inertia are miminum values with axes through the centroid. The parallel axes rule can be used to determine moments of inertia relative to displaced axes of rotation.

k ..Radius of Gyration

The radius with reference to an axis is that distance form the axis at which the entire mass of a body may be considered as concentrating keeping the moment of inertia unchanged.   

I = m.k_o²

Parallel Axis Rule

To determine the Moment of Inertia about an axis which is parallel to a centroid axis and at a distance d .

I_w = I + m.d²

Notes

C_x = centroid from yz plane....... C_y = centroid from xz plane....... C_z = centroid from xy plane..
I _xx, I _yy,I _zz are the principal mass moments of inertia for axes through the centroid C in the direction x,y, & z.

	Area Volume	Cx Cy Cz	I xx	I yy	I zz
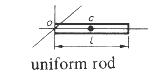	-- --	l /2 , 0, 0	0	m.l 2 / 12	m.l 2 / 12
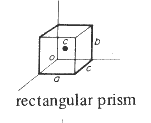	2.(a.b + a.c +b.c) a.b.c	a/2 , b/2 , c/2	m. (b 2 + c2 ) /12	m. (c 2 + a 2 ) /12	m. (a 2 + b2 ) / 12
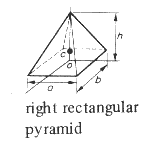	--- a.b.h /3	0, h/4, 0	m (4.b 2 + 3.h 2 ) /80	m (a 2 + b 2 ) /20	m .(4.a 2 + 3.h 2 ) /80
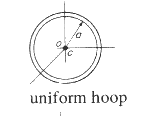	0 0	0, 0, 0	m.a 2 /2	m.a 2 /2	m.a 2
	4.π .a2 0	0, 0, 0	2.m.a 2 /3
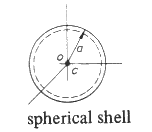
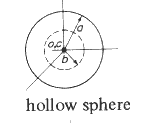	4.π .a 2 4.π. (a 3 - b 3 ) / 3	0, 0, 0	2.m.(a 5 - b 5) / 5.(a 3 - b 3)
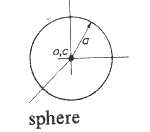	4.π .a2 4.π .a 3 /3	0 , 0, 0	2.m.a 2 /5
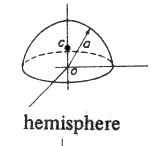	2.π.a2 2.π .a 3 /3	0, 3.a /8, 0	--	2.m.a2 /5	--
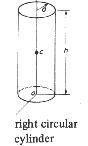	2.π.a.( h + a ) π.a 2 .h	0, h/2 , 0	m.(3.a 2 + h 2 )/12	m.a 2 /2	m.(3.a 2 + h 2 )/12
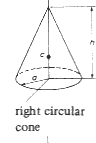	π.a.(Sqrt(a 2 + h 2)+a) π.a2 .h /3	0, h/4 , 0	3.m.(4.a 2 + h 2 )/80	3. m.a 2 / 10	3.m.(4.a 2 + h 2 )/80
	--- 2.π.a.b.c /3	0 , 0 , 0	m.(b 2 + c 2 )/5	m.(c 2 + a 2 )/5	m.(a 2 + b 2 )/5
	2.π.r.h	0, h/2, 0	--	--	--
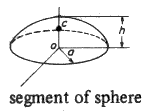a = radius of sphere	2. π.r.h π.h2 (r - h/3)	0 , h.(4.a -h)/ 4.(3.a - h), 0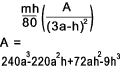

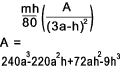Links to Properties of Figures Wolfram - M.of I. of common solids..High quality information Efunda - Common solids..Very clear comprehensive information on all important properties of solid shapes Scienceworld.wolfram .... Welcome to the best resource of Science and math on the Internet- Probably true Hyperphysics - Moment on Inertia..This is an excellent site for useful information

Maths Index