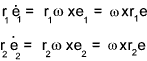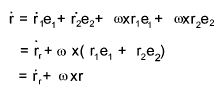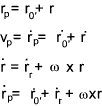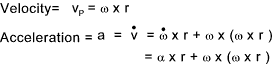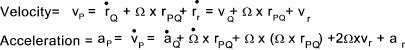| |
Mechanics Index
Introduction to Kinematics
|
Introduction Kinematics deals with the motion of bodies and requires consideration of the geometry and time. The forces
involved in the motion of body are not considered in this area of work. Basics
Nomenclature
Particle Kinematics.
This discipline of mechanics deals with the displacement of particles over time without
reference to the forces that cause the motion, velocity and acceleration of the particle. Linear Motion..
Considering a particle moving along a path is space from position A to position B over a time interval.
The position vector r locates the particle relative to the reference frame (say cartesian xyz) . The
distance the particle moves along the path is designated s.
The change in position of the particle over the time is its linear displacement and
is identified by the vector ( r B - r A ) Textbook formulae..Linear motion subject to uniform acceleration.
v = u + at
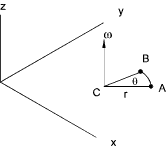
Angular Motion.. A point rotates from A to B around a center C through a small angle θ in a time t . As the time interval approaches zero the instantaneous angular velocity d θ /dt = ω . This angular velocity is a vector cross product and is shown in the direction as indicated below..
The velocity of the point is obtained as a vector product of the angular velocity and the radius v = ω x r Angular velocity vectors are free vectors but they are not commutative.. Textbook formulae.. Angular motion subject to uniform acceleration
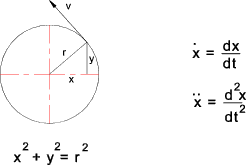
ω = 2.π .n /60 Motion in a circle.. A particle moving in a circle of radius (r) at a constant velocity (v) and constant
angular velocity of ω = v /r is accelerating towards the centre
of the circle at a constant rate of v 2 /r = ω 2 . r. The acceleration is primarily due to the rate of
change of angular position of the particle... An example of linear motion is that of a particle moving at a fixed velocity in a circular motion..
Differentiate with respect to time
Differentiate again with respect to time using ( d(uv) = u.dv + v du )
Relating the Tangential velocity to the x an y velocity.
Introducing velocity into equation
Finally establishing formula for accelaration
Absolute and relative motion.. A reference frame can be fixed or relative. In Newtonian mechanics the fixed reference frame is the primary inertial
system in which there is zero absolute motion in space. This is entirely impractical
because it would have to take into account the movements of the earth relative to the sun and the
sun in the universe etc. A reference frame based on the sun is called a Heliocentric reference frame
and this again is impractical for normal mechanical engineering. A practical reference
frame is one based on the earth. This is called a geocentric reference frame and is sufficient as an
inertial frame for normal engineering. Therefore when we identify the velocity of a vehicle or the
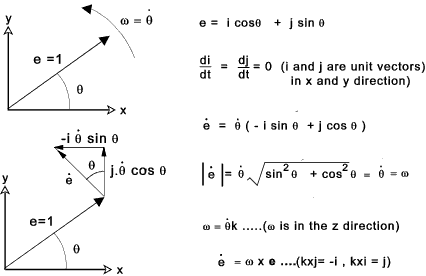
acceleration of an object they are generally relative to the earths motion. Rotating unit vector Consider a rotating unit vector e..
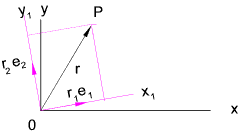
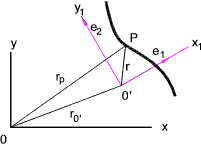
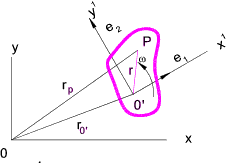
Vector of Point in a rotating reference frame A point P with a vector position r is moving in the xy plane. The position of this vector is identified in respect to unit vectors e 1 and e 2 fixed to a reference frame rotating at and angular velocity ω .. r 1 and r 2 are components of the motion in the e 1 and e 2 directions.
The position is expressed as follows..
The derivative of this equation with respect to time results in.
It is shown above that de/dt = ω x e therefore
Rewriting the equation
Velocity of a point in a moving reference frame A point P moving in the xy frame as shown below has a position vector of r p = r o' + r ro' locates O' relative to the fixed xy axes and r locates the point P relative to a axes (x 1,x 2)
If the moving frame is not rotating ω = 0
Differentiating (with respect to time) the equation for velocity (v) results the equation for accelaration (a)
Following the principles established above...
Note : The term .....
Rigid Body Kinematics in one plane.
Rigid body..A body with all internal points fixed relative to each other. Three
co-ordinates are required to determine the position and orientation of a body in plane motion.
A rigid body has three degrees of free in plane motion.
Plane motion for a rigid body can be easily developed from the equations for
motion of a particle. They are the same equations for the motion of a
point but as all points are fixed relative to each other the term involving
velocities and accelerations of points relative to each other are zero.
The equations for velocity and acceleration when the reference axes x',y' are fixed to the rigid body ,are as follows...
Important Note:
It can be easily proved that whichever position on the rigid body is selected for the
reference position O' the angular velocity and angular acceleration vectors are
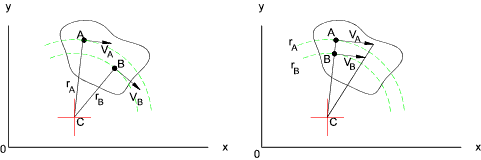
not changed. Instantaneous Centre of Rotation For any body moving in space the motion can be defined from the position, velocity, acceleration
of any point on the body with the angular velocity and acceleration. There is a point
for this body for which the instantaneous translational velocity is zero i.e only rotation of the body
about the point is occuring. This point is called the instantaneous center of rotation and
its location relative to the body is dependent on the relative values of the linear and angular velocities
For near zero angular velocities (near translation motion) the location is at near infinity.
The location of the instantaneous centre of rotation is easy to locate if the velocity and
angular velocity of a point are known Rigid Body kinematics in 3 Dimensions Introduction A particle requires three points to specify its position relative to the selected
coordinate system and is therefore identified as having 3 degrees of freedom
. For a rigid body the location of three separate points of the body relative
to the selected co-ordinate system are required. Because the points are
fixed relative to each other only six independent co-ordinates are required to locate
the body is space and a unrestrained rigid body is therefore said to have six degrees
of freedom.
e.g.
A rigid body may be positioned by locating the position of one point of the body ( 3 co-ordinates), then
positioning a line on the body (2 co-ordinates) and finally identifying a rotation about
the line (1 co-odinates). This sums up to 6 co-ordinates
Euler's Theorem Any displacement of a rigid body may be compounded from a single rotation about any
selected point plus a translation of that point. Any finite displacement of a rigid body may be reduced to a single rotation about an
axis plus a translation parallel to the same axis. This theorem only relates
the displacement and not to the paths taken by points. Any motion of a rigid body can be described by a single angular velocity plus a translational velocity parallel to the angular velocity vector. Any motion of a body about a point may be represented by a single angular velocity about an axis through that point. Any motion of a rigid body my be represented by the velocity of a point plus the angular velocity about an axis passing through the points... Rectilinear Translation.& Curvilinear Translation
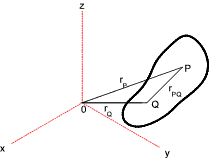
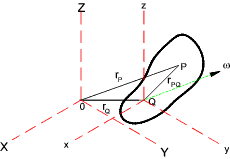
All points on the body will move in parallel straight or curve lines. There is no
relative velocity or acceleration between any points on the body. Position... r P = r Q + r PQ Velocity...v P = v Q + v PQ Rotation about a fixed axis.
Rotation of a rigid body about fixed axis is shown in the diagram.
The vector of the rotary motion has sense and direction in accordance with the right hand rule
which is fixed along the direction of the axis. There is zero velocity
due to rotation at the axis.
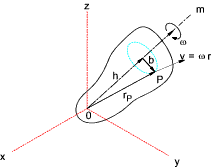
Rotation about a fixed Point. Rotation of a rigid body about a fixed point can always be reduced to rotation of a
body about an instantaneous axis of rotation.
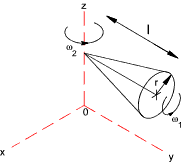
If ω2 = 0 then the axis rotation is the centre line of the shaft
and the velocity of any point on the shaft is proportional to the radius (max = r)from
the shaft. If the rotation about the vertical axis is increased to a certain
value the the velocity of the shaft axis is proportional to ω2 x
the shaft length (max = l) from the axis (radius).
If the two angular velocities are the same and the radius of the cone r = l then
at any instant the velocity of top surface of the cone is zero. i.e the top surface is
the instantaneous axis of rotation.
ω = ω1 + ω2 = ω1i + ω2j In the real world the motions and shapes are not as shown but the interaction between
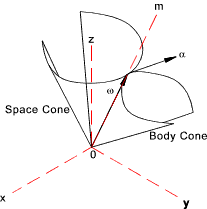
the body rotation on its axis and its angular motion is space still results in an instantaneous
axis of rotation between a instantaneous body cone and an instantaneous space cone.
The body cone may rotate outside or inside the space cone
One determining the instantaneous angular velocity the angular acceleration α is tangential to the contact point of the two cones as shown. The velocity and acceleration at any point is simply determined as shown below
General Motion. The general case of 3D motion of rigid bodies reduces to translation + rotation about
a fixed axis. This is basically a generalisation of the theorems provided above.
r P = r Q + r PQ
The above equations are sufficient if the angular velocity ω is zero however if this is not the case the following equations for velocity and acceleration are more definitive;
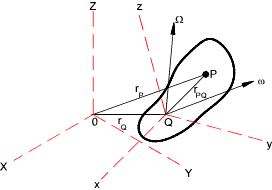
Rotating reference system A more general form of the relative reference axis method uses rotating reference
axes. The reference axes xyz are rotating with an angular velocity of Ω. The rigid body
has a rotation velocity ω as before
The basic motion equations are as above. The expression for velocity and acceleration of point
P are provided below. The derivation is a simple extension of that provided above for 2
Dimensional motion with a rotating relative axis with the third dimension (z) added.
v r and a r = are the velocity and acceleration of P relative to the rotating xyz axis. Note: The equations above are based on the general case of the angular velocity of the rigid body (ω)is different to the angular velocity of the axis ( Ω). If the reference axis is fixed to the body then ω will equal Ω and vr and a r will equal zero... For this case the formula will be the same as for the translating reference axis above.. |
Links to Kinematics
|
|
Mechanics Index
Send Comments to