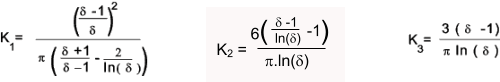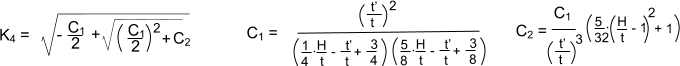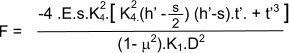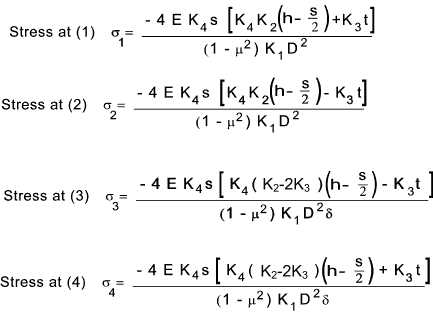Machine_Parts_Index
Spring Index Disc Spring Design
More details on Spring design are to be found in the links below the table.
|
Introduction A disc spring is a conical shell spring which is loaded along its
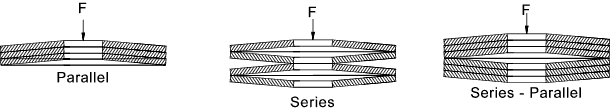
axis. Disc springs can used as single or multiple units. When stacked in multiple units
they can be stacked in series to give a low stiffness value or in parallel to give a higher stiffness value.
By varying the size and the stacking arrangements an extremely wide variation in operating parameters
can be achieved.
Parallel Stacked springs (n springs)..For a given force the spring deflection will be (1/n) x the
deflection of a single spring. The stress experienced by each spring will be 1/n the stress experienced by the single spring.
(friction must be considered when loading is constantly changing )
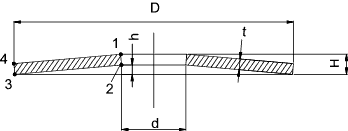
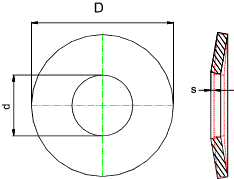
Nomenclature
Factors The factors are calculated as follows.
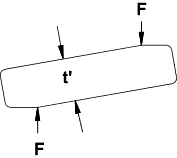
Springs With Contact Surfaces Some of the springs in group 2 and all of the springs in group 3 are manufactured with contact surfaces to enable better load bearing. These flats provide improved contact between springs and they also reduce the outside diameter. A consequence of the altered
geometry is that higher forces are generated. To compensate for this undesirable effect
the thickness of the spring is reduced from t to t'.
A factor K4 is provided to allow for the different operating characteristics for disc springs with contact surfaces.
If the disc
spring has no contact surfaces the K4 = 1 Spring Force For Goup 1 and Group 2 disc springs without contact surfaces (see note below) K4 = 1 The force at a given disc spring deflection is obtained by the formula below. This is for
springs with no contact surfaces
For springs Group 3 disc spring with contact surfaces the formula below is more accurate. When considering springs with contact surfaces. Use the factor K4 as calculated
below and use t' instead of t and use h' = H - t'
Spring Stresses The stresses in a disc spring at four critical locations 1,2,3,4 see sketch for positions
are shown below _-ve values are compressive stresses and +ve values are tensile stresses)
|
Links to Spring Design
|
|
Machine_Parts_Index
Spring Index