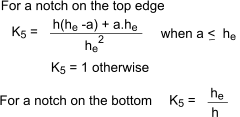Timber Modification Factors

Modification Factors used in Timber Calculations

Introduction

The timber calculations reviewed in the timber design pages on this website are based on BS EN 5288 Part 2. This standards includes modification factors used to allow for operating conditions and loading scenarios. This page provides some guidance in assessing these factors

Relevant Standards..For comprehensive list of standards Wood related Standards

BS 5268 -2 ;2002 Structural use of timber � Part 2: Code of practice for permissible stress design, materials and workmanship.

Modifying factors

K₂ by which stresses and moduli for service classes 1 and 2 should be multiplied to obtain stresses and modulii applicable to service class 3
K₃ for duration of loading
K₄ for bearing stress
K₅ for notched beams
K₆ for shapes other than rectangular
K₇ width factor for bending stresses for widths other than 300mm
K₈ for load sharing applications
K₉ used to modify the minimum modulus of elasticity for trimmer joists and lintels
K₁₂ for compression members
K₁₃ for the effective length of spaced columns
K₁₄ width factor for tensile loaded members for widths other than 300mm
K15, K16, K17, K18, K19 and K20 for single grade glued laminated members and horizontally
K27, K28 and K29 for vertically glued laminated members
K30, K31 and K32 for individually designed glued end joints in horizontally glued laminated members

Information on correction factors

K₂

Modification factor, K2, by which stresses and moduli for service classes 1 and 2 should be multiplied to obtain stresses and moduli applicable to service class 3.

Note:.. The properties provided in BS 5368:2007 for the different timbers are generally applicable to Service class 1 & 2. Please refer to notes on service class Service Class

Property	Value of K₂
Bending parallel to grain	0,8
Tension parallel to grain	0,8
Compression paralle to grain	0,6
Compression parallel to grain	0,6
Shear parallel to grain	0,9
Mean & Min Modulus of Elasticiy	0,8

K₃

Duration of Load	Value of K₃
Long Term (e.g.permanently imposed )	1,00
Medium term (e.g. dead + temporary imposed	1,25
Short Term (e.g. dead + imposed + wind	1,5
Very short term (e.g. dead + wind	1,75

K₄

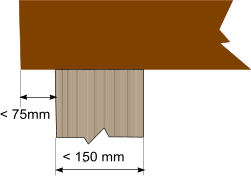
The grade stresses for compression perpendicular to the grain apply to bearings of any length at the ends of a member, and bearings 150 mm or more in length at any position. However for bearings less than 150 mm long located 75 mm or more from the end of a member, as shown in figure belwo thee grade stress should be multiplied by the modification factor, K4

length of Bearing	Value of K₄
10	1,74
15	1,67
25	1,53
40	1,33
50	1,20
75	1,14
100	1,10
150+	1,00

K₅

This factor is applied for members taking load at notched ends as shown below

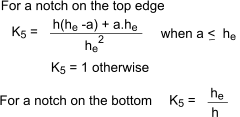
K₆

Grade bending stresses primarily apply to solid timber members of rectangular cross-section. For other shapes of cross-section the grade bending stresses should be multiplied by the modification factor, K6, where

K₆ = 1.18 for solid circular sections;
K₆ = 1.41 for solid square sections loaded on a diagonal.

K₇

The grade bending stresses provided in the tables is generally based on sections 300mm deep a constant (K₇)is used when other thicknesses are used.

K₈

In construction applications where four or more members are sharing the imposed load e.g. rafters , joists, trusses etc. with suitable provisions for distributing the load e.g. boarding, battens etc the admissibles stresses and the modulus shoul be obtained using the following rules .

The appropriate grade stresses should be multiplied by the load sharing modification factor, K8 = 1.1.
The mean modulus of elasticity should be used to calculate deflections and displacements under both dead and imposed load. However if the loading results from mechanical plant and equipment, for storage, or for floors subject to vibrations, then the minimum modulus of elasticity should be used.

K₉

The members which are sharing the load, as described above, the minimum modulus of elasticity hould be modified by the factor, K9, when calculating the deflection deflections

No Pieces	Value of K₉
No Pieces	Softwoods	Hardwoods
1	1,00	1,00
2	1,14	1,06
3	1,21	1,08
4 or more	1,24	1,10

K₁₄

The grade compressive stress listed in the tables are based on a width of 300mm. For timber members of other sizes a factor K₁₄ should be used.

K₁₄ = 1,17 for solid timber with a width less than 72mm

K₁₄ = (300 h.)^0,11 for solid members having a width h greater than 72mm

K₁₅,K₁₆,K₁₇K₁₈,K₁₉,K₁₉

Modifcation factors for horizontally glued single grade laminates

Strength Class	Number of Laminations	Bending Parallel to Grain	Tension parallel to grain	Compression parallel to grain	Compression normal to grain	Shear parallel to grain>	Modulus of Elasticity
Strength Class	Number of Laminations	K₁₅	K₁₆	K₁₇	K₁₈	K₁₉	K₂₀
C27,C30,C35, C40 D50,D60,D70	4 or More	1,1	1,1	1,04	1,49	1,49	1,00
C16,C18,C22, C24 D30,D35,D40	4	1,26	1,26	1,04	1,55	2,34	1,07
	5	1,34	1,34
	7	1,39	1,39
	10 or more	1,42	1,42

K₂₇,K₂₈,K₂₉

Modification factors for vertically glued single grade laminates

Number of laminations	Bending, Tension and shear parallel to grain K₂₇		Mod of elasticity and compression parallel to grain K₂₈		Compression normal to grain K₂₉
Number of laminations	Softwoods	Hardwoods	Softwoods	Hardwoods	Softwoods and Hardwoods
2	1,11	1,06	1,14	1,06	1,10
3	1,16	1,08	1,21	1,08	1,10
4	1,19	1,10	1,24	1,10	1,10
5	1,21	1,11	1,27	1,11	1,10
6	1,23	1,12	1,29	1,12	1,10
7	1,24	1,12	1,30	1,12	1,10

K₃₀,K₃₁,K₃₂

Timber	Bending, parallel to grain	Tension, parallel to grain	Compression, parallel to grain
Timber	K₂₈	K₃₁	K₃₂
Softwood	1,63	1,63	1,43
Hardwood	1,32	1,32	1,42

Links Providing relevant informationd

Matter Index Page
Timber Index Page