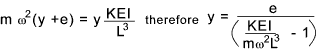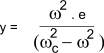Drive_Index
Critical (Whirling) Speed Of Shafts
|
Introduction For a rotating shaft there is a speed at which, for any small initial
deflection, the centripetral force is equal to the elastic restoring force. At this
point the deflection increases greatly and the shaft is said to "whirl". Below and above this speed
this effect is very much reduced. This critical (whirling speed) is dependent on
the shaft dimensions, the shaft material and the shaft loads . The critical speed is
the same as the frequency of traverse vibrations. Where m = the mass of the shaft assumed concentrated at single point . For a horizontal shaft this can be expressed as
Where y = the static deflection at the location of the concentrated mass Symbols
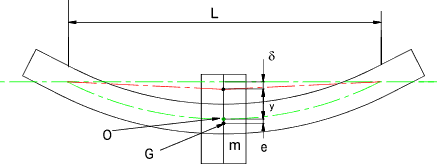
Theory Consider a rotating horizontal shaft with a central mass (m) which has a centre of gravity (G)slightly away from the geometric centroid(O)
The centrifugal force on the shaft = m ω 2(y + e) and the inward pull exerted by
the shaft = y (48EI / L 3).
When the denominator = 0 ,that is [ KEI / m ω 2 L 3 ] = 1 ,
the deflection becomes infinite and whirling takes place.
For a simply supported beam with a central mass K = 48 .. See examples below Substituting ω c 2 for KEI /mL3 in the above equation for y results in the following equation which relates the angular velocity with the deflection.
This is plotted below..
This curve shows the deflection of the shaft (from the static deflection position) at any speed ω in terms of the critical speed. Cantilever rotating mass Mass of shaft neglected
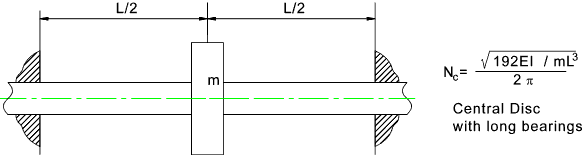
Central rotating mass- Long Bearings Mass of shaft neglected
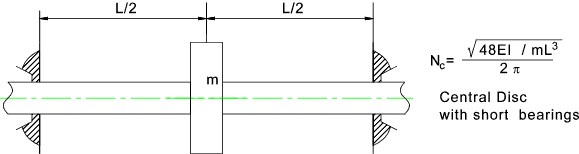
Central rotating mass - Short Bearings Mass of shaft neglected
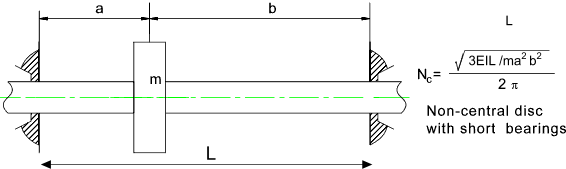
Non-Central rotating mass - Short Bearings Mass of shaft neglected
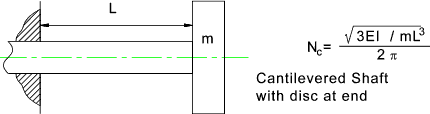
Cantilevered Shaft
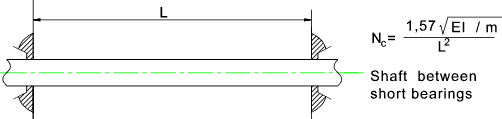
m = mass /unit length Shaft Between short bearings
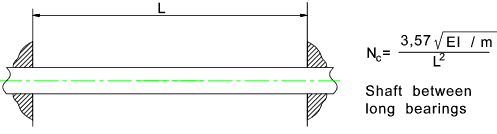
m = mass /unit length Shaft Between long bearings
m = mass /unit length Combined loading This is known as Dunkerley's method an is based on the theory of superposition....
|
Links to Information on designing shaft suppliers and shaft locking methods
|
|
Drive_Index