Note:
Components which are subject to continuously cyclic loading often fail prematurely as a result of fatigue.  The worst fatigue loading regimes are loads which continuously reverse from negative (compressive) loading to positive (tensile) loading in a cyclic manner. Reference fatigue loading notes  Fatigue Index

As springs are often used under continuously fluctuating loading conditions it is necessary to consider fatigue loading and stress concentration factors.  Helical springs are never used under conditions of load reversals.   They are either normally in tension or normally in compression.  In addition springs are often prestressed as part of the forming process or/and preloaded, thus preventing the stress from being zero.  These factors mitigate, to some extent, the fatigue loading conditions.

All spring subject to continuous fluctuating load are candidates for fatigue failure. Typical springs are

Nomenclature

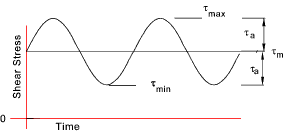
Fatigue Notes

The normal shear stress condition experienced be a spring subject to continuous fluctuating loading is as shown below

The force amplitude and mean value are calculated as

The resulting alternating and mean stresses are

For springs the safety factor for torsional endurance life is

Experimental results have proved that for spring steel the torsional endurance limit is not directly related to size, tensile strength, or material for wires under 10mm diameter.   The resulting value from experiments has been determined as

S'_se = 310 MPa for unpeened springs and 465 MPa for peened springs

S'_sa = 241 MPa for unpeened springs and 398 MPa for peened springs

S'_sm = 379 MPa for unpeened springs and 534 MPa for peened springs

These values include all modifying factors except for the reliability factor. ref Fatigue modifying factors That is S_e = C_rS'_e

For springs subject to low cyclic /static loading the safety factor for torsional yielding is

It is generally safe to use a torsional yield strength of 40% of the ultimate tensile strength i.e S_syof 0,4S_ut ref notes Spring Materials

If the spring applications between 10³ and 10⁶ cycles of variation a modified torsional shear strength ( S_fs )can be used to determine the safety margin.

S_sf is the modified shear fatigue strength. This can be determined approximately if the endurance limit( S'_se ) and the fatigue strength at 10³ cycles ( S'_sl ) are available ref High cycle fatigue strength

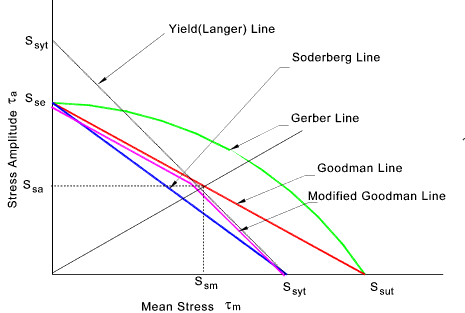
The fatigue design of springs generally involves one of a number of failure criterions, as shown below.

The intersect equation for the Goodmans criterion is

The relevant factor of safety is calculated as follows