|
Introduction These initial notes relate to single degree of freedom systems under the influence
of periodic excitation forces i.e.the force is sinusoidal in nature.
If the solution of this general case is known then, in principle, other more complicated
period forces can be determined by superimposing the effects of as many sinusoidal components
as required. Other force types e.g. rotating unbalanced forces
will be addressed later. Forced Vibration
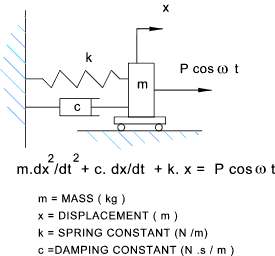
The equation of motion for the above system is m . dx2 /dt 2 + c . dx /dt + k . x = P cos ω t The general solution of the equation is the sum of two parts x = xc + xp
The amplitude of the complementary solution decreases with time and is therefore
a transient part of the general solution. Notes on solving the complementary solution are provided on
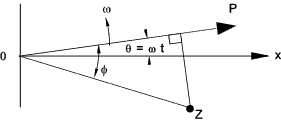
webpage Free vibrations e iθ = cosθ + i sin θ putting ω t in place of θ e iω t = cosω t + i sin ω t Note: cos ω t is the real component of ei ω t. Substitution of the above into the Euler formula results in the equation xp = Real( A e i (ω t - φ)) = Real ( A e -i φ e i ω t ) Next substitute Z for the complex constant A e -iφ The modulus of
Z is the amplitude of the steady state solution . The argument of Z is φ, the phase
of the steady state solution.
Referring to the initial equation
a) xp = Real ( Z e i ω t ) Substituting these values into the original equation Real [ ( -m ω2 + i c ω + k ) Z e i ω t ] = Real ( P e i ω t ) The solution for x is provided if P = ( -m ω2 + i c ω + k ) Z 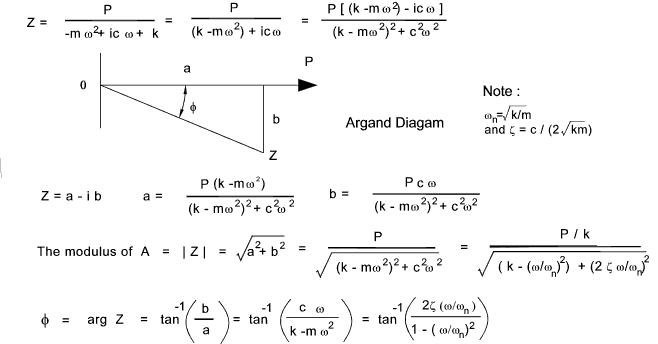 The steady state solution is therefore: x = A cos ( ω t - φ ) |
Links on Forced Vibration
|
|