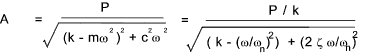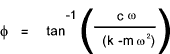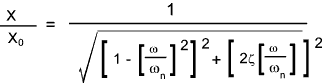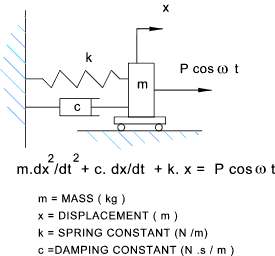
On the page   Ref..Forced Vibrations    covering vibration of a mass (m) with a k spring stiffness and c viscous damping subject to a sinusoidal force of P cosω t.

The steady state solution for the system was shown to be

x = A cos ( ω t - φ )

The modulus A was shown to be

The phase angle φ was shown to be

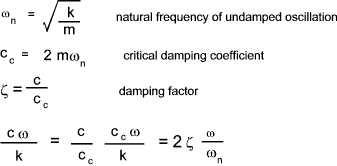
using the following derived relationships..
.

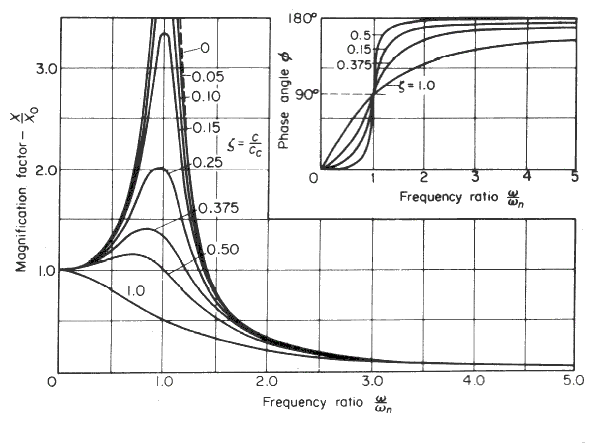
The dimensionless relationship between the Static displacement P/k (= X_o )and the Peak amplitude X is provided by the following expression

The phase angle can be related to the driving frequency and the natural frequency

For the case of a mass M supported on springs k with viscous damping c which is supporting a rotating unbalanced load of mass m rotating with and angular frequency ω at a radius r .    The peak amplitude and phase are provided by the following dimensionless expressions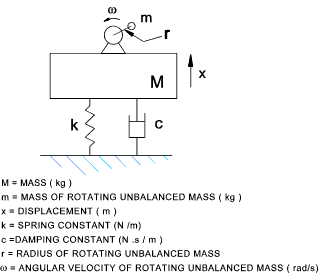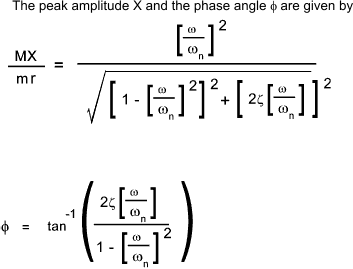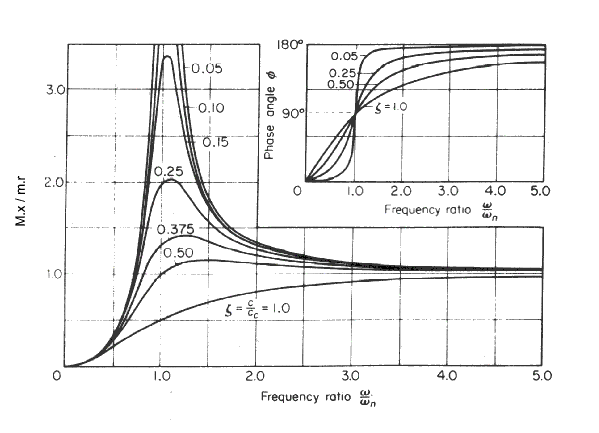

For the case of a mass m supported on springs k with viscous damping c on a base which is moving with an excitation of form y = Y sin ω t .


Note: proof may be most easily be obtained from link 3 below;

The ratio of peak ampltude of the mass and the base and the phase are provided by the following dimensionless expressions

Note: The amplitude ratio is also the ratio of the transmitted force to the exciting force it is a measure of the transmissibility of the system � the ratio of the energy going into the system to the energy coming from the system.