The study of forces resulting from the impact of fluid jets and when fluids are diverted round pipe bends involves the application of newtons second law in the form of F = m.a. The forces are determined by calculating the change of momentum of the flowing fluids.    In nature these forces manifest themselves in the form of wind forces, and the impact forces of the sea on the harbour walls.   The operation of hydro-kinetic machines such as turbines depends on forces developed through changing the momentum of flowing fluids.

I have created a excelcalcs spreadsheet for convenient access to all of the equations found on this page . This is located at ExcelCalcs.com calculation Fluid Jets

Newtons Second Law can be stated as: The force acting on a body in a fixed direction is equal to rate of increase of momentum of the body in that direction.  Force and momentum are vector quantities so the direction is important. 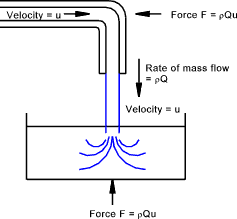A fluid is essentially a collection of particles and the net force, in a fixed direction, on a defined quantity of fluid equals the total rate of momentum of that fluid quantity in that direction.

Consider a mass m which has an initial velocity u and is brought to rest. Its loss of momentum is m.u and if it stopped in a time interval t then the rate of change of momentum is m.u /t.    The force F required to stop the moving mass is therefore F = m.u / t .  Now if this is applied to a jet of fluid with a mass flow rate ( m / t ) which is equivalent to the volumetric flow rate times the density ( Qρ ) the equivalent force on a flowing fluid is F = Qρ u.  Also in accordance with Newtons third law the resulting force of the fluid by a flowing fluid on its surroundings is (-F).   Newtons third law states that for every force there is an equal and opposite force.

The figure below illustrates this principle at two locations.

The fluid flowing into the tank is brought to rest from a velocity u to zero velocity and the force on the jet is F = Q.ρ. u. .  The reaction force on the tank contents is -F.

The fluid flowing in the pipe in the horizontal direction is forced to change direction at the bend such that its velocity in the orginal direction is zero.  Therefore the force on the flowing fluid is F = Q. ρ u. The reaction force on the pipe is -F in the horizontal direction as shown.

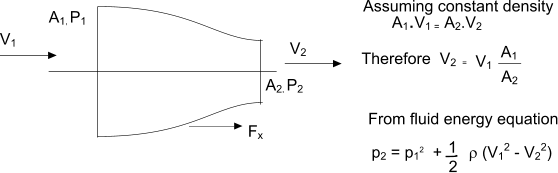
In its simplest form, with steady flow conditions, the force on a fluid flow in a set direction is equal to its mass flow rate times by the change in velocity in the set direction.   The fluid flow also exerts an equal and opposite reaction force as a result of this change in momentum.

F = Qρ (u ₁ - u ₂). ..( F and u are vector quantities)

The resultant force on a fluid in a particular direction is equal to the rate of increase of momentum in that direction.

Jet force on a flat plate

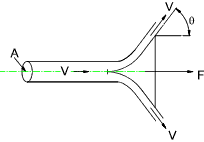
Considering only forces in a horizontal direction u ₁ = V and u ₂ = 0 therefore
F = QρV = ρAV ²

Jet force on a flat plate at an angle θ

Considering only forces Normal to plate surface u ₁ = V sinθ and u ₂ = 0 therefore
F = QρV sin θ = ρAV ² sin θ

when θ =90^o then F = ρAV ² as above

Jet force on an angled plate. (θ < 90 ^o)

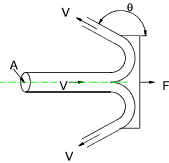
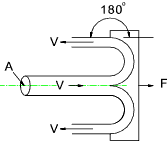
Considering only forces in a horizontal direction u ₁ = V and u ₂ = V cos θ therefore
F = QρV (1 - cosθ ) = ρAV ²(1 - cosθ )

Jet Force on an angled plate (θ > 90 ^o)

Considering only forces in a horizontal direction u ₁ = V and u ₂ = V cos θ therefore
F = QρV (1 - cosθ ) = ρAV ²(1 - cosθ )

Jet Force on an angled plate (θ = 180 ^o)

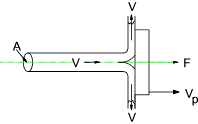
u ₁ = V and u ₂ = V cos θ therefore
F = QρV (1 - cos180 ^o )   =   2QρV   =   2ρAV ²

Jet force on a moving flat plate

Considering only forces in a horizontal direction
u ₁ = V and u ₂ = V p
and let r = V _p / V therefore
F = Qρ( V - V _p ) = ρAV(V -V _p)
and F = ρAV ²( 1 - r

The power (P) generated by the force on the moving plate =      P = F. V p

Jet force on an angled moving plate

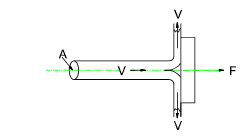
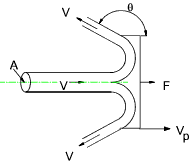
Considering only forces in a horizontal direction
u ₁ = V and u ₂ = V _p + (V - V _p) cos θ
and let r = V _p / V therefore
F = ρA V( V - V _p ) ( 1 - cosθ ) = ρA V ² ( 1 - r ) ( 1 - cosθ )
The power (P) generated by the force on the moving plate       P = F. V p

Note: The moving plate with an angle θ = 180^o is the typical of the rotating plate of the pelton wheel.
The ideal value for r resulting in the maximum power output is clearly 0,5

Additional notes can be found on webpages
Fluid Machines - Francis Wheel
Steam Turbines - Impulse blades

Force on fixed Vane.

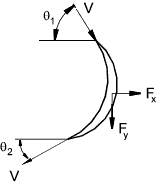
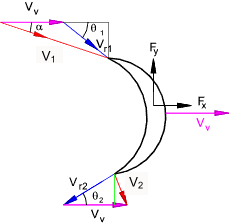
In the x Direction: u _1x = V cos θ ₁ ,     u _2x = -V cos θ ₂
F _x = QρV(cos θ ₁ + cos θ ₂ ) = ρAV ²(cos θ ₁ + cos θ ₂ )

In the y Direction u _1y = V sin θ ₁ u _2y = V sin θ ₂
F _y = QρV(sin θ ₁ - sin θ ₂ ) = ρAV ²(sin θ ₁ - sin θ ₂ )

Force on Moving Vane.

The notes below related to vanes as used in impulse turbines.  These turbines derive the mechanical energy mainly from the change in momentum as the fluid passes through the vanes.    The conditions as shown when the vectorial sum of V _v + V _r1 = V₁ results in smooth entry with efficient transfer of energy of the fluid to the vane.     When this does not occur there will be turbulent flow over the vane with significant losses.

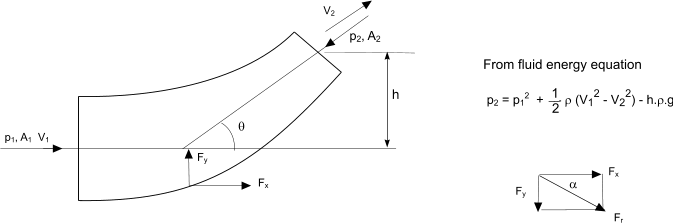
In the x Direction
u _1x = V ₁ cos α
u _2x = V _v - V _r2 cos θ ₂  .... ( V _r2 = V _r1 = V ₁ sin α /sin θ ₁ )
F _x = QρV ₁ (cos α + [sin α /sin θ ₁ ] cos θ ₂ - r > )
r = V _v / V ₁

In the y Direction u _1y = V ₁ sin α
u _2y = V _r2 sin θ ₂  .... ( V _r2 = V _r1 = V ₁ sin α /sin θ ₁ )
In the y direction F _y = QρV ₁sin α (1 - sin θ ₂ / sin θ ₁ )

If the vane is moving in the x direction the power developed by the vane P = F _x.V _V

Force on Pipe Wall

The notes below related to the force on a pipe wall resulting from the changes in fluid pressure and fluid momentum as the fluid flows round a pipe bend . Gravity and friction effects are not considered.    The fluid is assumed to be flowing under steady state conditions.

In the x Direction

u ₁ = V ₁
u ₂ = V ₂
F _x = p₁.A₁ - p₂.A₂ + ρ (A₁V₁² - A₂V₂)²

The resultant reaction force on the pipe = F_r =√ (F_x² + F_x² )
The angle α of the resultant force to the x axis = tan^-1 ((F_y /(F_x)