Introduction
When comparing two samples it is often necessary to test the validity that the samples are from
the same distribution. The F ratio test is used for this purpose.
The F-test is used to test if the standard deviations of two populations
are equal or if one the standard deviation from one population is less than that of another population.
This test can be a two-tailed test or a one-tailed test. The two-tailed
version tests against the alternative that the standard deviations are not equal.
The one-tailed version only tests in one direction, that is the standard deviation from
the first population is either greater than or less than (but not both) the second population standard
deviation. One - Two tailed tests
The standard deviation is directly related to the precision so
if it needed to test if measuring process is more precise than
another the F - test is ideal.
It is necessary to recognise that the F-test is only robust if the populations under
study have normal probability distributions. If the populations are not normal
then the F-test is unreliable.
Symbols
α = Significance (1% 5% etc)
γ = Confidence ( 99% etc)
n = sample number
f(x) = probability function. (values between 0 and 1)
F(x) = probability distribution function.
ν = number of degrees of freedoms
Xm = Sample mean
var = sample variance
|
Φ (x) = Probability distribution function.(Standardised probability )
μ = population /random variable mean
σ 2 = population /random variable variance
σ = population /random variable standard deviation
xm = arithmetic mean of sample
sx 2 = variance of sample
sx = Standard deviation of sample
|
Degrees of Freedom
The number of degrees of freedom as applicable in the F -test
ν = n - 1
Examples
Example 1:
To test if one method (Method A) of measuring the density a fluid is more accurate than
a second method (Method B). Each method was tested Eleven times, yielding
the following values:
| Method | Mean (kg/m2 | Standard Deviation (kg/m2 |
| A | 600 | 9 |
| B | 800 | 13 |
A method is more precise if its standard deviation σ is
lower so it is necessary to test the null hypotheses that the variances are equal
(Ho:σ B 2 = σ A 2 ) against the
alternative hypothesis that the variance of method A is less than the variance of method B(H1: σ B 2 > σ A 2)
The degrees of freedom ν = n - 1 = 10 in each case.
Since σ B > σ A then
F calc = [ σ B2 / σ A2 ] = 169 /81 = 2,086.
With both ν = 10 and using the one table values below for confidence values results in an F10,10 value 2,978. The calculated F value is less than
the tabled value 2,086 < 2,978 and therefore the null hypothesis that both methods are as precise is accepted and the
alternative H1 hypothesis that σ B > σ B is not accepted
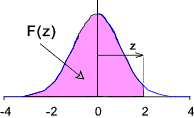
F- Distribution with (m,n) degrees of Freedom..With F(z) = 0,95
| n |
m |
| 1 |
2 |
3 |
4 |
5 |
6 |
7 |
8 |
9 |
10 |
12 |
24 |
25 |
30 |
50 |
100 |
500 |
5000 |
| 1 |
161,45 |
199,5 |
215,71 |
224,58 |
230,16 |
233,99 |
236,77 |
238,88 |
240,54 |
241,88 |
243,9 |
249,05 |
249,26 |
250,1 |
251,77 |
253,04 |
254,06 |
254,29 |
| 2 |
18,51 |
19 |
19,16 |
19,25 |
19,3 |
19,33 |
19,35 |
19,37 |
19,38 |
19,4 |
19,41 |
19,45 |
19,46 |
19,46 |
19,48 |
19,49 |
19,49 |
19,5 |
| 3 |
10,13 |
9,55 |
9,28 |
9,12 |
9,01 |
8,94 |
8,89 |
8,85 |
8,81 |
8,79 |
8,74 |
8,64 |
8,63 |
8,62 |
8,58 |
8,55 |
8,53 |
8,53 |
| 4 |
7,71 |
6,94 |
6,59 |
6,39 |
6,26 |
6,16 |
6,09 |
6,04 |
6 |
5,96 |
5,91 |
5,77 |
5,77 |
5,75 |
5,7 |
5,66 |
5,64 |
5,63 |
| 5 |
6,61 |
5,79 |
5,41 |
5,19 |
5,05 |
4,95 |
4,88 |
4,82 |
4,77 |
4,74 |
4,68 |
4,53 |
4,52 |
4,5 |
4,44 |
4,41 |
4,37 |
4,37 |
| 6 |
5,99 |
5,14 |
4,76 |
4,53 |
4,39 |
4,28 |
4,21 |
4,15 |
4,1 |
4,06 |
4 |
3,84 |
3,83 |
3,81 |
3,75 |
3,71 |
3,68 |
3,67 |
| 7 |
5,59 |
4,74 |
4,35 |
4,12 |
3,97 |
3,87 |
3,79 |
3,73 |
3,68 |
3,64 |
3,57 |
3,41 |
3,4 |
3,38 |
3,32 |
3,27 |
3,24 |
3,23 |
| 8 |
5,32 |
4,46 |
4,07 |
3,84 |
3,69 |
3,58 |
3,5 |
3,44 |
3,39 |
3,35 |
3,28 |
3,12 |
3,11 |
3,08 |
3,02 |
2,97 |
2,94 |
2,93 |
| 9 |
5,12 |
4,26 |
3,86 |
3,63 |
3,48 |
3,37 |
3,29 |
3,23 |
3,18 |
3,14 |
3,07 |
2,9 |
2,89 |
2,86 |
2,8 |
2,76 |
2,72 |
2,71 |
| 10 |
4,96 |
4,1 |
3,71 |
3,48 |
3,33 |
3,22 |
3,14 |
3,07 |
3,02 |
2,98 |
2,91 |
2,74 |
2,73 |
2,7 |
2,64 |
2,59 |
2,55 |
2,54 |
| 11 |
4,84 |
3,98 |
3,59 |
3,36 |
3,2 |
3,09 |
3,01 |
2,95 |
2,9 |
2,85 |
2,79 |
2,61 |
2,6 |
2,57 |
2,51 |
2,46 |
2,42 |
2,41 |
| 12 |
4,75 |
3,89 |
3,49 |
3,26 |
3,11 |
3 |
2,91 |
2,85 |
2,8 |
2,75 |
2,69 |
2,51 |
2,5 |
2,47 |
2,4 |
2,35 |
2,31 |
2,3 |
| 13 |
4,67 |
3,81 |
3,41 |
3,18 |
3,03 |
2,92 |
2,83 |
2,77 |
2,71 |
2,67 |
2,6 |
2,42 |
2,41 |
2,38 |
2,31 |
2,26 |
2,22 |
2,21 |
| 14 |
4,6 |
3,74 |
3,34 |
3,11 |
2,96 |
2,85 |
2,76 |
2,7 |
2,65 |
2,6 |
2,53 |
2,35 |
2,34 |
2,31 |
2,24 |
2,19 |
2,14 |
2,13 |
| 15 |
4,54 |
3,68 |
3,29 |
3,06 |
2,9 |
2,79 |
2,71 |
2,64 |
2,59 |
2,54 |
2,48 |
2,29 |
2,28 |
2,25 |
2,18 |
2,12 |
2,08 |
2,07 |
| 16 |
4,49 |
3,63 |
3,24 |
3,01 |
2,85 |
2,74 |
2,66 |
2,59 |
2,54 |
2,49 |
2,42 |
2,24 |
2,23 |
2,19 |
2,12 |
2,07 |
2,02 |
2,01 |
| 17 |
4,45 |
3,59 |
3,2 |
2,96 |
2,81 |
2,7 |
2,61 |
2,55 |
2,49 |
2,45 |
2,38 |
2,19 |
2,18 |
2,15 |
2,08 |
2,02 |
1,97 |
1,96 |
| 18 |
4,41 |
3,55 |
3,16 |
2,93 |
2,77 |
2,66 |
2,58 |
2,51 |
2,46 |
2,41 |
2,34 |
2,15 |
2,14 |
2,11 |
2,04 |
1,98 |
1,93 |
1,92 |
| 19 |
4,38 |
3,52 |
3,13 |
2,9 |
2,74 |
2,63 |
2,54 |
2,48 |
2,42 |
2,38 |
2,31 |
2,11 |
2,11 |
2,07 |
2 |
1,94 |
1,89 |
1,88 |
| 20 |
4,35 |
3,49 |
3,1 |
2,87 |
2,71 |
2,6 |
2,51 |
2,45 |
2,39 |
2,35 |
2,28 |
2,08 |
2,07 |
2,04 |
1,97 |
1,91 |
1,86 |
1,84 |
| 21 |
4,32 |
3,47 |
3,07 |
2,84 |
2,68 |
2,57 |
2,49 |
2,42 |
2,37 |
2,32 |
2,25 |
2,05 |
2,05 |
2,01 |
1,94 |
1,88 |
1,83 |
1,81 |
| 22 |
4,3 |
3,44 |
3,05 |
2,82 |
2,66 |
2,55 |
2,46 |
2,4 |
2,34 |
2,3 |
2,23 |
2,03 |
2,02 |
1,98 |
1,91 |
1,85 |
1,8 |
1,78 |
| 23 |
4,28 |
3,42 |
3,03 |
2,8 |
2,64 |
2,53 |
2,44 |
2,37 |
2,32 |
2,27 |
2,2 |
2,01 |
2 |
1,96 |
1,88 |
1,82 |
1,77 |
1,76 |
| 24 |
4,26 |
3,4 |
3,01 |
2,78 |
2,62 |
2,51 |
2,42 |
2,36 |
2,3 |
2,25 |
2,18 |
1,98 |
1,97 |
1,94 |
1,86 |
1,8 |
1,75 |
1,73 |
| 25 |
4,24 |
3,39 |
2,99 |
2,76 |
2,6 |
2,49 |
2,4 |
2,34 |
2,28 |
2,24 |
2,16 |
1,96 |
1,96 |
1,92 |
1,84 |
1,78 |
1,73 |
1,71 |
| 26 |
4,23 |
3,37 |
2,98 |
2,74 |
2,59 |
2,47 |
2,39 |
2,32 |
2,27 |
2,22 |
2,15 |
1,95 |
1,94 |
1,9 |
1,82 |
1,76 |
1,71 |
1,69 |
| 27 |
4,21 |
3,35 |
2,96 |
2,73 |
2,57 |
2,46 |
2,37 |
2,31 |
2,25 |
2,2 |
2,13 |
1,93 |
1,92 |
1,88 |
1,81 |
1,74 |
1,69 |
1,67 |
| 28 |
4,2 |
3,34 |
2,95 |
2,71 |
2,56 |
2,45 |
2,36 |
2,29 |
2,24 |
2,19 |
2,12 |
1,91 |
1,91 |
1,87 |
1,79 |
1,73 |
1,67 |
1,66 |
| 29 |
4,18 |
3,33 |
2,93 |
2,7 |
2,55 |
2,43 |
2,35 |
2,28 |
2,22 |
2,18 |
2,1 |
1,9 |
1,89 |
1,85 |
1,77 |
1,71 |
1,65 |
1,64 |
| 30 |
4,17 |
3,32 |
2,92 |
2,69 |
2,53 |
2,42 |
2,33 |
2,27 |
2,21 |
2,16 |
2,09 |
1,89 |
1,88 |
1,84 |
1,76 |
1,7 |
1,64 |
1,62 |
| 31 |
4,16 |
3,3 |
2,91 |
2,68 |
2,52 |
2,41 |
2,32 |
2,25 |
2,2 |
2,15 |
2,08 |
1,88 |
1,87 |
1,83 |
1,75 |
1,68 |
1,62 |
1,61 |
| 32 |
4,15 |
3,29 |
2,9 |
2,67 |
2,51 |
2,4 |
2,31 |
2,24 |
2,19 |
2,14 |
2,07 |
1,86 |
1,85 |
1,82 |
1,74 |
1,67 |
1,61 |
1,6 |
| 33 |
4,14 |
3,28 |
2,89 |
2,66 |
2,5 |
2,39 |
2,3 |
2,23 |
2,18 |
2,13 |
2,06 |
1,85 |
1,84 |
1,81 |
1,72 |
1,66 |
1,6 |
1,58 |
| 34 |
4,13 |
3,28 |
2,88 |
2,65 |
2,49 |
2,38 |
2,29 |
2,23 |
2,17 |
2,12 |
2,05 |
1,84 |
1,83 |
1,8 |
1,71 |
1,65 |
1,59 |
1,57 |
| 35 |
4,12 |
3,27 |
2,87 |
2,64 |
2,49 |
2,37 |
2,29 |
2,22 |
2,16 |
2,11 |
2,04 |
1,83 |
1,82 |
1,79 |
1,7 |
1,63 |
1,57 |
1,56 |
| 36 |
4,11 |
3,26 |
2,87 |
2,63 |
2,48 |
2,36 |
2,28 |
2,21 |
2,15 |
2,11 |
2,03 |
1,82 |
1,81 |
1,78 |
1,69 |
1,62 |
1,56 |
1,55 |
| 37 |
4,11 |
3,25 |
2,86 |
2,63 |
2,47 |
2,36 |
2,27 |
2,2 |
2,14 |
2,1 |
2,02 |
1,82 |
1,81 |
1,77 |
1,68 |
1,62 |
1,55 |
1,54 |
| 38 |
4,1 |
3,24 |
2,85 |
2,62 |
2,46 |
2,35 |
2,26 |
2,19 |
2,14 |
2,09 |
2,02 |
1,81 |
1,8 |
1,76 |
1,68 |
1,61 |
1,54 |
1,53 |
| 39 |
4,09 |
3,24 |
2,85 |
2,61 |
2,46 |
2,34 |
2,26 |
2,19 |
2,13 |
2,08 |
2,01 |
1,8 |
1,79 |
1,75 |
1,67 |
1,6 |
1,53 |
1,52 |
| 40 |
4,08 |
3,23 |
2,84 |
2,61 |
2,45 |
2,34 |
2,25 |
2,18 |
2,12 |
2,08 |
2 |
1,79 |
1,78 |
1,74 |
1,66 |
1,59 |
1,53 |
1,51 |
| 41 |
4,08 |
3,23 |
2,83 |
2,6 |
2,44 |
2,33 |
2,24 |
2,17 |
2,12 |
2,07 |
2 |
1,79 |
1,78 |
1,74 |
1,65 |
1,58 |
1,52 |
1,5 |
| 42 |
4,07 |
3,22 |
2,83 |
2,59 |
2,44 |
2,32 |
2,24 |
2,17 |
2,11 |
2,06 |
1,99 |
1,78 |
1,77 |
1,73 |
1,65 |
1,57 |
1,51 |
1,49 |
| 43 |
4,07 |
3,21 |
2,82 |
2,59 |
2,43 |
2,32 |
2,23 |
2,16 |
2,11 |
2,06 |
1,99 |
1,77 |
1,76 |
1,72 |
1,64 |
1,57 |
1,5 |
1,49 |
| 44 |
4,06 |
3,21 |
2,82 |
2,58 |
2,43 |
2,31 |
2,23 |
2,16 |
2,1 |
2,05 |
1,98 |
1,77 |
1,76 |
1,72 |
1,63 |
1,56 |
1,49 |
1,48 |
| 45 |
4,06 |
3,2 |
2,81 |
2,58 |
2,42 |
2,31 |
2,22 |
2,15 |
2,1 |
2,05 |
1,97 |
1,76 |
1,75 |
1,71 |
1,63 |
1,55 |
1,49 |
1,47 |
| 46 |
4,05 |
3,2 |
2,81 |
2,57 |
2,42 |
2,3 |
2,22 |
2,15 |
2,09 |
2,04 |
1,97 |
1,76 |
1,75 |
1,71 |
1,62 |
1,55 |
1,48 |
1,46 |
| 47 |
4,05 |
3,2 |
2,8 |
2,57 |
2,41 |
2,3 |
2,21 |
2,14 |
2,09 |
2,04 |
1,96 |
1,75 |
1,74 |
1,7 |
1,61 |
1,54 |
1,47 |
1,46 |
| 48 |
4,04 |
3,19 |
2,8 |
2,57 |
2,41 |
2,29 |
2,21 |
2,14 |
2,08 |
2,03 |
1,96 |
1,75 |
1,74 |
1,7 |
1,61 |
1,54 |
1,47 |
1,45 |
| 49 |
4,04 |
3,19 |
2,79 |
2,56 |
2,4 |
2,29 |
2,2 |
2,13 |
2,08 |
2,03 |
1,96 |
1,74 |
1,73 |
1,69 |
1,6 |
1,53 |
1,46 |
1,45 |
| 50 |
4,03 |
3,18 |
2,79 |
2,56 |
2,4 |
2,29 |
2,2 |
2,13 |
2,07 |
2,03 |
1,95 |
1,74 |
1,73 |
1,69 |
1,6 |
1,52 |
1,46 |
1,44 |
| 100 |
3,94 |
3,09 |
2,7 |
2,46 |
2,31 |
2,19 |
2,1 |
2,03 |
1,97 |
1,93 |
1,85 |
1,63 |
1,62 |
1,57 |
1,48 |
1,39 |
1,31 |
1,29 |
| 150 |
3,9 |
3,06 |
2,66 |
2,43 |
2,27 |
2,16 |
2,07 |
2 |
1,94 |
1,89 |
1,82 |
1,59 |
1,58 |
1,54 |
1,44 |
1,34 |
1,25 |
1,23 |
| 200 |
3,89 |
3,04 |
2,65 |
2,42 |
2,26 |
2,14 |
2,06 |
1,98 |
1,93 |
1,88 |
1,8 |
1,57 |
1,56 |
1,52 |
1,41 |
1,32 |
1,22 |
1,19 |
| 500 |
3,86 |
3,01 |
2,62 |
2,39 |
2,23 |
2,12 |
2,03 |
1,96 |
1,9 |
1,85 |
1,77 |
1,54 |
1,53 |
1,48 |
1,38 |
1,28 |
1,16 |
1,12 |
| 5000 |
3,84 |
3 |
2,61 |
2,37 |
2,22 |
2,1 |
2,01 |
1,94 |
1,88 |
1,83 |
1,75 |
1,52 |
1,51 |
1,46 |
1,35 |
1,25 |
1,11 |
1,05 |
F- Distribution with (m,n) degrees of Freedom..With F(z) = 0,99

| n |
m |
| 1 |
2 |
3 |
4 |
5 |
6 |
7 |
8 |
9 |
10 |
12 |
24 |
25 |
30 |
50 |
100 |
500 |
5000 |
| 1 |
4052 |
4999 |
5403 |
5624 |
5763 |
5859 |
5928 |
5981 |
6022 |
6056 |
6107 |
6234 |
6240 |
6260 |
6302 |
6334 |
6360 |
6366 |
| 2 |
98,5 |
99 |
99,16 |
99,25 |
99,3 |
99,33 |
99,36 |
99,38 |
99,39 |
99,4 |
99,42 |
99,46 |
99,46 |
99,47 |
99,48 |
99,49 |
99,5 |
99,5 |
| 3 |
34,12 |
30,82 |
29,46 |
28,71 |
28,24 |
27,91 |
27,67 |
27,49 |
27,34 |
27,23 |
27,05 |
26,6 |
26,58 |
26,5 |
26,35 |
26,24 |
26,15 |
26,13 |
| 4 |
21,2 |
18 |
16,69 |
15,98 |
15,52 |
15,21 |
14,98 |
14,8 |
14,66 |
14,55 |
14,37 |
13,93 |
13,91 |
13,84 |
13,69 |
13,58 |
13,49 |
13,47 |
| 5 |
16,26 |
13,27 |
12,06 |
11,39 |
10,97 |
10,67 |
10,46 |
10,29 |
10,16 |
10,05 |
9,89 |
9,47 |
9,45 |
9,38 |
9,24 |
9,13 |
9,04 |
9,02 |
| 6 |
13,75 |
10,92 |
9,78 |
9,15 |
8,75 |
8,47 |
8,26 |
8,1 |
7,98 |
7,87 |
7,72 |
7,31 |
7,3 |
7,23 |
7,09 |
6,99 |
6,9 |
6,88 |
| 7 |
12,25 |
9,55 |
8,45 |
7,85 |
7,46 |
7,19 |
6,99 |
6,84 |
6,72 |
6,62 |
6,47 |
6,07 |
6,06 |
5,99 |
5,86 |
5,75 |
5,67 |
5,65 |
| 8 |
11,26 |
8,65 |
7,59 |
7,01 |
6,63 |
6,37 |
6,18 |
6,03 |
5,91 |
5,81 |
5,67 |
5,28 |
5,26 |
5,2 |
5,07 |
4,96 |
4,88 |
4,86 |
| 9 |
10,56 |
8,02 |
6,99 |
6,42 |
6,06 |
5,8 |
5,61 |
5,47 |
5,35 |
5,26 |
5,11 |
4,73 |
4,71 |
4,65 |
4,52 |
4,41 |
4,33 |
4,31 |
| 10 |
10,04 |
7,56 |
6,55 |
5,99 |
5,64 |
5,39 |
5,2 |
5,06 |
4,94 |
4,85 |
4,71 |
4,33 |
4,31 |
4,25 |
4,12 |
4,01 |
3,93 |
3,91 |
| 11 |
9,65 |
7,21 |
6,22 |
5,67 |
5,32 |
5,07 |
4,89 |
4,74 |
4,63 |
4,54 |
4,4 |
4,02 |
4,01 |
3,94 |
3,81 |
3,71 |
3,62 |
3,6 |
| 12 |
9,33 |
6,93 |
5,95 |
5,41 |
5,06 |
4,82 |
4,64 |
4,5 |
4,39 |
4,3 |
4,16 |
3,78 |
3,76 |
3,7 |
3,57 |
3,47 |
3,38 |
3,36 |
| 13 |
9,07 |
6,7 |
5,74 |
5,21 |
4,86 |
4,62 |
4,44 |
4,3 |
4,19 |
4,1 |
3,96 |
3,59 |
3,57 |
3,51 |
3,38 |
3,27 |
3,19 |
3,17 |
| 14 |
8,86 |
6,51 |
5,56 |
5,04 |
4,69 |
4,46 |
4,28 |
4,14 |
4,03 |
3,94 |
3,8 |
3,43 |
3,41 |
3,35 |
3,22 |
3,11 |
3,03 |
3,01 |
| 15 |
8,68 |
6,36 |
5,42 |
4,89 |
4,56 |
4,32 |
4,14 |
4 |
3,89 |
3,8 |
3,67 |
3,29 |
3,28 |
3,21 |
3,08 |
2,98 |
2,89 |
2,87 |
| 16 |
8,53 |
6,23 |
5,29 |
4,77 |
4,44 |
4,2 |
4,03 |
3,89 |
3,78 |
3,69 |
3,55 |
3,18 |
3,16 |
3,1 |
2,97 |
2,86 |
2,78 |
2,76 |
| 17 |
8,4 |
6,11 |
5,19 |
4,67 |
4,34 |
4,1 |
3,93 |
3,79 |
3,68 |
3,59 |
3,46 |
3,08 |
3,07 |
3 |
2,87 |
2,76 |
2,68 |
2,66 |
| 18 |
8,29 |
6,01 |
5,09 |
4,58 |
4,25 |
4,01 |
3,84 |
3,71 |
3,6 |
3,51 |
3,37 |
3 |
2,98 |
2,92 |
2,78 |
2,68 |
2,59 |
2,57 |
| 19 |
8,18 |
5,93 |
5,01 |
4,5 |
4,17 |
3,94 |
3,77 |
3,63 |
3,52 |
3,43 |
3,3 |
2,92 |
2,91 |
2,84 |
2,71 |
2,6 |
2,51 |
2,49 |
| 20 |
8,1 |
5,85 |
4,94 |
4,43 |
4,1 |
3,87 |
3,7 |
3,56 |
3,46 |
3,37 |
3,23 |
2,86 |
2,84 |
2,78 |
2,64 |
2,54 |
2,44 |
2,42 |
| 21 |
8,02 |
5,78 |
4,87 |
4,37 |
4,04 |
3,81 |
3,64 |
3,51 |
3,4 |
3,31 |
3,17 |
2,8 |
2,79 |
2,72 |
2,58 |
2,48 |
2,38 |
2,36 |
| 22 |
7,95 |
5,72 |
4,82 |
4,31 |
3,99 |
3,76 |
3,59 |
3,45 |
3,35 |
3,26 |
3,12 |
2,75 |
2,73 |
2,67 |
2,53 |
2,42 |
2,33 |
2,31 |
| 23 |
7,88 |
5,66 |
4,76 |
4,26 |
3,94 |
3,71 |
3,54 |
3,41 |
3,3 |
3,21 |
3,07 |
2,7 |
2,69 |
2,62 |
2,48 |
2,37 |
2,28 |
2,26 |
| 24 |
7,82 |
5,61 |
4,72 |
4,22 |
3,9 |
3,67 |
3,5 |
3,36 |
3,26 |
3,17 |
3,03 |
2,66 |
2,64 |
2,58 |
2,44 |
2,33 |
2,24 |
2,21 |
| 25 |
7,77 |
5,57 |
4,68 |
4,18 |
3,85 |
3,63 |
3,46 |
3,32 |
3,22 |
3,13 |
2,99 |
2,62 |
2,6 |
2,54 |
2,4 |
2,29 |
2,19 |
2,17 |
| 26 |
7,72 |
5,53 |
4,64 |
4,14 |
3,82 |
3,59 |
3,42 |
3,29 |
3,18 |
3,09 |
2,96 |
2,58 |
2,57 |
2,5 |
2,36 |
2,25 |
2,16 |
2,13 |
| 27 |
7,68 |
5,49 |
4,6 |
4,11 |
3,78 |
3,56 |
3,39 |
3,26 |
3,15 |
3,06 |
2,93 |
2,55 |
2,54 |
2,47 |
2,33 |
2,22 |
2,12 |
2,1 |
| 28 |
7,64 |
5,45 |
4,57 |
4,07 |
3,75 |
3,53 |
3,36 |
3,23 |
3,12 |
3,03 |
2,9 |
2,52 |
2,51 |
2,44 |
2,3 |
2,19 |
2,09 |
2,07 |
| 29 |
7,6 |
5,42 |
4,54 |
4,04 |
3,73 |
3,5 |
3,33 |
3,2 |
3,09 |
3 |
2,87 |
2,49 |
2,48 |
2,41 |
2,27 |
2,16 |
2,06 |
2,04 |
| 30 |
7,56 |
5,39 |
4,51 |
4,02 |
3,7 |
3,47 |
3,3 |
3,17 |
3,07 |
2,98 |
2,84 |
2,47 |
2,45 |
2,39 |
2,25 |
2,13 |
2,03 |
2,01 |
| 31 |
7,53 |
5,36 |
4,48 |
3,99 |
3,67 |
3,45 |
3,28 |
3,15 |
3,04 |
2,96 |
2,82 |
2,45 |
2,43 |
2,36 |
2,22 |
2,11 |
2,01 |
1,98 |
| 32 |
7,5 |
5,34 |
4,46 |
3,97 |
3,65 |
3,43 |
3,26 |
3,13 |
3,02 |
2,93 |
2,8 |
2,42 |
2,41 |
2,34 |
2,2 |
2,08 |
1,98 |
1,96 |
| 33 |
7,47 |
5,31 |
4,44 |
3,95 |
3,63 |
3,41 |
3,24 |
3,11 |
3 |
2,91 |
2,78 |
2,4 |
2,39 |
2,32 |
2,18 |
2,06 |
1,96 |
1,94 |
| 34 |
7,44 |
5,29 |
4,42 |
3,93 |
3,61 |
3,39 |
3,22 |
3,09 |
2,98 |
2,89 |
2,76 |
2,38 |
2,37 |
2,3 |
2,16 |
2,04 |
1,94 |
1,91 |
| 35 |
7,42 |
5,27 |
4,4 |
3,91 |
3,59 |
3,37 |
3,2 |
3,07 |
2,96 |
2,88 |
2,74 |
2,36 |
2,35 |
2,28 |
2,14 |
2,02 |
1,92 |
1,89 |
| 36 |
7,4 |
5,25 |
4,38 |
3,89 |
3,57 |
3,35 |
3,18 |
3,05 |
2,95 |
2,86 |
2,72 |
2,35 |
2,33 |
2,26 |
2,12 |
2 |
1,9 |
1,87 |
| 37 |
7,37 |
5,23 |
4,36 |
3,87 |
3,56 |
3,33 |
3,17 |
3,04 |
2,93 |
2,84 |
2,71 |
2,33 |
2,31 |
2,25 |
2,1 |
1,98 |
1,88 |
1,86 |
| 38 |
7,35 |
5,21 |
4,34 |
3,86 |
3,54 |
3,32 |
3,15 |
3,02 |
2,92 |
2,83 |
2,69 |
2,32 |
2,3 |
2,23 |
2,09 |
1,97 |
1,86 |
1,84 |
| 39 |
7,33 |
5,19 |
4,33 |
3,84 |
3,53 |
3,3 |
3,14 |
3,01 |
2,9 |
2,81 |
2,68 |
2,3 |
2,29 |
2,22 |
2,07 |
1,95 |
1,85 |
1,82 |
| 40 |
7,31 |
5,18 |
4,31 |
3,83 |
3,51 |
3,29 |
3,12 |
2,99 |
2,89 |
2,8 |
2,66 |
2,29 |
2,27 |
2,2 |
2,06 |
1,94 |
1,83 |
1,81 |
| 41 |
7,3 |
5,16 |
4,3 |
3,81 |
3,5 |
3,28 |
3,11 |
2,98 |
2,87 |
2,79 |
2,65 |
2,28 |
2,26 |
2,19 |
2,04 |
1,92 |
1,82 |
1,79 |
| 42 |
7,28 |
5,15 |
4,29 |
3,8 |
3,49 |
3,27 |
3,1 |
2,97 |
2,86 |
2,78 |
2,64 |
2,26 |
2,25 |
2,18 |
2,03 |
1,91 |
1,8 |
1,78 |
| 43 |
7,26 |
5,14 |
4,27 |
3,79 |
3,48 |
3,25 |
3,09 |
2,96 |
2,85 |
2,76 |
2,63 |
2,25 |
2,23 |
2,17 |
2,02 |
1,9 |
1,79 |
1,77 |
| 44 |
7,25 |
5,12 |
4,26 |
3,78 |
3,47 |
3,24 |
3,08 |
2,95 |
2,84 |
2,75 |
2,62 |
2,24 |
2,22 |
2,15 |
2,01 |
1,89 |
1,78 |
1,75 |
| 45 |
7,23 |
5,11 |
4,25 |
3,77 |
3,45 |
3,23 |
3,07 |
2,94 |
2,83 |
2,74 |
2,61 |
2,23 |
2,21 |
2,14 |
2 |
1,88 |
1,77 |
1,74 |
| 46 |
7,22 |
5,1 |
4,24 |
3,76 |
3,44 |
3,22 |
3,06 |
2,93 |
2,82 |
2,73 |
2,6 |
2,22 |
2,2 |
2,13 |
1,99 |
1,86 |
1,76 |
1,73 |
| 47 |
7,21 |
5,09 |
4,23 |
3,75 |
3,43 |
3,21 |
3,05 |
2,92 |
2,81 |
2,72 |
2,59 |
2,21 |
2,19 |
2,12 |
1,98 |
1,85 |
1,74 |
1,72 |
| 48 |
7,19 |
5,08 |
4,22 |
3,74 |
3,43 |
3,2 |
3,04 |
2,91 |
2,8 |
2,71 |
2,58 |
2,2 |
2,18 |
2,12 |
1,97 |
1,84 |
1,73 |
1,71 |
| 49 |
7,18 |
5,07 |
4,21 |
3,73 |
3,42 |
3,19 |
3,03 |
2,9 |
2,79 |
2,71 |
2,57 |
2,19 |
2,18 |
2,11 |
1,96 |
1,83 |
1,72 |
1,7 |
| 50 |
7,17 |
5,06 |
4,2 |
3,72 |
3,41 |
3,19 |
3,02 |
2,89 |
2,78 |
2,7 |
2,56 |
2,18 |
2,17 |
2,1 |
1,95 |
1,82 |
1,71 |
1,69 |
| 100 |
6,9 |
4,82 |
3,98 |
3,51 |
3,21 |
2,99 |
2,82 |
2,69 |
2,59 |
2,5 |
2,37 |
1,98 |
1,97 |
1,89 |
1,74 |
1,6 |
1,47 |
1,43 |
| 150 |
6,81 |
4,75 |
3,91 |
3,45 |
3,14 |
2,92 |
2,76 |
2,63 |
2,53 |
2,44 |
2,31 |
1,92 |
1,9 |
1,83 |
1,66 |
1,52 |
1,38 |
1,34 |
| 200 |
6,76 |
4,71 |
3,88 |
3,41 |
3,11 |
2,89 |
2,73 |
2,6 |
2,5 |
2,41 |
2,27 |
1,89 |
1,87 |
1,79 |
1,63 |
1,48 |
1,33 |
1,28 |
| 500 |
6,69 |
4,65 |
3,82 |
3,36 |
3,05 |
2,84 |
2,68 |
2,55 |
2,44 |
2,36 |
2,22 |
1,83 |
1,81 |
1,74 |
1,57 |
1,41 |
1,23 |
1,17 |
| 5000 |
6,64 |
4,61 |
3,79 |
3,32 |
3,02 |
2,81 |
2,64 |
2,51 |
2,41 |
2,32 |
2,19 |
1,79 |
1,78 |
1,7 |
1,53 |
1,36 |
1,16 |
1,07 |
|
