Thermodynamics & Heat Transfer

This page provides a limited notes on thermodyamics and heat transfer that may be useful to mechanical engineers.

Definition

Thermodynamics ..From the Greek thermos meaning heat and dynamis meaning power.

Thermodynamics covers the relationship between heat, work, temperature, and energy, including the general behaviour of physical systems in a condition of equilibrium or close to it. It is a fundamental part of all the physical sciences.

Thermodynamics is an experimental science based on a small number of principles that are derived from experience. Classical Thermodynamics is concerned only with macroscopic or large-scale properties of matter and does not include the study of small-scale or microscopic structure of matter. Statistical Thermodynamics ( not covered on this website) includes the study of the thermal relationships of atomic sized particles

Notation

Identifier	Description	Units (typical)
c _p	Specific Heat Capacity at Constant pressure	kJ/(kg K)
c _v	Specific Heat Capacity at Constant Volume	kJ/(kg K)
p	Absolute Pressure	N / m ²
T	Absolute Temperature	K
v	volume per unit mass	m ³
W	Work Output per unit mass	kJ/kg
M	Molecular Weight	-
R _o	Universal Gas Constant = 8,31	kJ /(kg mole.K)
Q	Heat Quantity	kJ
R	Gas Constant = R _o / M	kJ /kg.K
U	Internal energy (thermal)	kJ

Thermodynamic Laws

Zeroth Law of Thermodynamics

When two objects are separately in thermodynamic equilibrium with a third they are in equilibrium with each other

Objects in thermodynamic equilibrium are at the same temperature

First Law of Thermodynamics...

This law expresses the general law of conservation of energy. and states that heat and work are mutually convertible

Heat In = Work Out over complete cycle
or Sum (d Q ) = sum (d W )

The basic energy equation results from this

dQ = dU + dW

Second Law of Thermodynamics

This law in its simplest states that heat can only flow from hot to cold and not vice versa. In terms of thermodynamic engine cycles the law states that the gross heat supplied to a system in a complete cycle must exceed the work done by the system. Therefore heat must be rejected. The thermal efficiency of an heat engine must be less than 100%.

Process Relations

Reversible Polytropic Process

p v ⁿ = constant

W = ( p ₂ v ₂ - p ₁ v ₁ ) / ( 1 - n ) .. (n not 0 )

For a perfect gas

W = R ( T ₂ - T ₁ ) / (1 -n )

Q = ( Cv + R /(1 - n) ) ( T ₂ -T ₁ )

T ₂ / T ₁ = ( p ₂ / p ₁ ) ^{( n-1 ) / n}

For Adiabatic processes (Q = 0 ) n = γ = cp / cv

γ = 1.4 for Air, H ₂, O ₂, CO, NO, Hcl

γ = 1.3 for CO ₂, SO ₂, H ₂O, H ₂S, N ₂O, NH ₃, CL ₂, CH ₄, C ₂H ₂, C ₂H ₄

Heat Transfer

Heat Transfer takes place by Conduction, Convection and Radiation

Heat Transfer by Conduction

q = Heat Flow Rate W
t ₁ & t ₂ = temperature, K (heat flows down (-))
A = Area, m ²
k = Coefficient of thermal conductivity, W m ^-1K ^-1
U = Overall Heat Transfer Coefficient,W m ^-1K ^-1
h = Surface Heat Transfer Coefficient, W m ^-2K ^-1
R = Thermal Resistance, W ^-1.m ^-1.K

dq = kA(-dt/dx)

q = (k.A /x). (t ₁-t ₂)

U = k/x

Therefore q = U.A(t ₁-t ₂)

Thermal resistance R = 1 / U.A

The heat has to pass through the surface layers on both sides of the wall

q = A.h _s1(t _s1 - t ₁) = k.A(t ₁ -t ₂) / x = Ah _s2(t ₂ -t _s2)

U = 1 / (1/h _s1 + x/k + 1/h _s2 )

R = 1/A.h _s1 + 1/A.h _s2 + x/A.k = R _s1 + R _s2 + R

Table Showing Various values for k at 20 ^oC


Metal	k=Wm^-1K^-1
Aluminium	237
Antimony	18.5
Beryllium	218
Brass	110
Cadmium	92
Cobalt	69
Constantan	22
Copper	398
Gold	315
Iridium	147
Cast Iron	55
Pure Iron	80.3
Wr't Iron	59
Lead	35.2
Magnesium	156
Molybdenum	138
Monel	26
Nickel	90.5
Platinum	73
Silver	427
C.Steel	50
St.Steel	25
Tin	67
Zinc	113

Plastics
Acrylic	0.2
Nylon 6	0.25;
Polythene High Den	0.5
PTFE	0.25
PVC	0.19


Misc.solids	k =Wm^-1K^-1
Asphalt	1.26
Bitumen	0.17
Br'ze Block	0.15
Brickwork	0.6
Brick-Dense	1.6
Carbon	1.7
Conc-LD	0.2
Conc-MD	0.5
Conc-HD	1.5
Firebrick	1.09
Glass	1.05
Glass -Boro.	1.3
Ice	2.18
Limestone	1.1
Mica	0.75
Cement	1.01
Parafin Wax	0.25
Porcelain	1.05
Sand	0.06

Insulation
Balsa	0.048
Straw-Comp	0.09
Cotton Wool	0.029
Polystyrene-Expanded	0.03
Felt	0.04
Glass Wool	0.04
Kapok	0.034
Magnesia	0.07
Plywood	0.13
Rock Wool	0.045
Sawdust	0.06
Slag Wool	0.042
Wood	0.13


Liquids	k= Wm^-1K^-1
Benzene	0.16
Carb Tet'ide	0.11
Acetone	0.16
Ether	0.14
Glycerol	0.28
Kerosene	0.15
Mercury	8
Methanol	0.21
Machine Oil	0.15
Water	0.58
Sodium	84

Gases	k= Wm ^-1K ^-1
Air	0.024
Ammonia	0.022
Argon	0.016
Carbon Dio	0.015
Carbon Mon	0.023
Helium	0.142
Hydrogen	0.168
Methane	0.030
Nitrogen	0.024
Oxygen	0.024
Water Vap.	0.016

Heat Transfer by Radiation

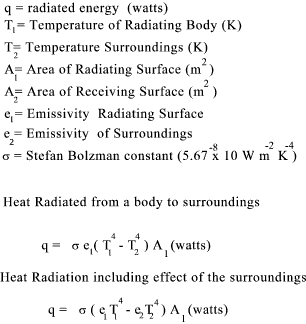
Emissivity Values

Refer to link Emissivity Values for better table

Surface Material	Emmissity	Surface Material	Emmissity
Aluminium-Oxidised	0.11	Tile	0.97
Aluminium-Polished	0.05	Water	0.95
Aluminium anodised	0.77	Wood-Oak	0.9
Aluminium rough	0.07	Paint	0.96
Asbestos Board	0.94	Paper	0.93
Black Body -Matt	1.00	Plastics	0.91 Av
Brass -Dull	0.22	Rubber-Nat_Hard	0.91
Brass- Polished	0.03	Rubber _Nat_Soft	0.86
Brick -Dark	0.9	Steel_Oxidised	0.79
Concrete	0.85	Steel Polished	0.07
Copper-Oxidised	0.87	St.Steel-Weathered	0.85
Copper -Polished	0.04	St.Steel-Polished	0.15
Glass	0.92	Steel Galv. Old	0.88
Plaster	0.98	Steel Galv new	0.23

Heat Transfer by Convection

Convective heat transfer occurs between a moving fluid and a solid surface.The rate of convective heat transfer between a surface and a fluid is given by the Newton�s Law of Cooling;

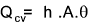
It is customary to express the convection coefficient (average or local), in a non-dimensional form called the Nusselt Number.

Natural convection

Nu = C(Gr.Pr) ⁿ C and n are tabled below

Note: Convection heat transfer values are very specific to the geometry of the surface and the heat transfer conditions - These example equations are very general in nature and should not be used for serious calcs. The links below provide much safer equations..

Surface	(Gr.Pr)	C	n
Vertical Plates/Cylinders	10 ⁴ to 10 ⁹	0.59	0.25
Vertical Plates/Cylinders	10 ⁹ to 10 ¹²	0.13	0.33
Horizontal Pipes	10 ³ to 10 ⁹	0.53	0.25
Horizontal Plates Heated Face up or Cooled Face Down	10 ⁵ to 2 x 10 ⁷	0.54	0.25
Horizontal Plates Heated Face up or Cooled Face Down	2 x10 ⁷ to 3 x10 ¹⁰	0.14	0.33
Horizontal Plates Heated Face up or Cooled Face Down	3 x10 ⁵ to 3 x10 ¹⁰	0.27	0.25

Forced Convection

Laminar flow over Plate Nu = 0.664(Re) ^1/2(Pr) ^1/3

Fully Developed pipe flow Nu = 0.0866(D/L)Re.Pr / (1+0.04[D / L(Re.Pr)] ^2/3) + 3.66

Turbulent Flow Over Flat Plate Nu = 0.036Pr ^1/3Re ^0.8

Turbulent Flow In Pipe Nu = 0.023Pr ^0.4Re ^0.8

Typical Values of Heat Transfer Coefficient h = W.m ^-2K ^-1

Free Convection Over Various Shape - Air 2 - 23
Free Convection Over Various Shape - Water 300 - 1700
Turbulent Convection Over Various Shape and through tubes - Air 6 - 1400
Turbulent Convection Over Various Shape and through tubes - Water 1100 - 9000

Heat Exchangers

Heat exchangers normally transfer energy from a hot fluid to a colder fluid. The energy in = The energy out.

If the fluids are the same with the same specific heat. The mass flowrate x the temp drop of the hot fluid = the mass flow rate x the temp rise of the cold fluid.

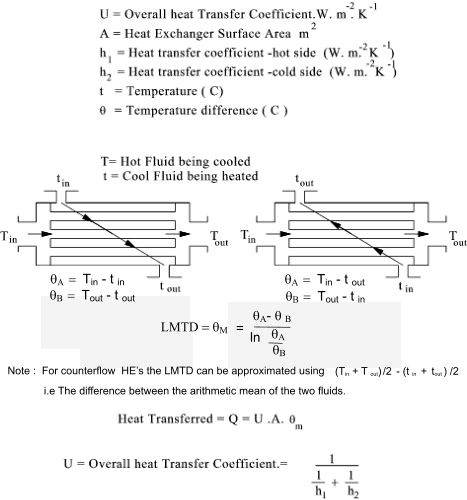
Typical Values for Overall Heat transfer U are

Plate Heat Exchanger, liquid to liquid U range 1000 > 4000 W. m.^-2K.^-1
Shell and Tube, liquid inside and outside tubes U range150 > 1200 W. m.^-2K.^-1.
Spiral Heat Exchanger, liquid to liquid U range 700 > 2500 W. m.^-2K.^-1